
Question and Answers Forum
Question Number 80792 by john santu last updated on 06/Feb/20
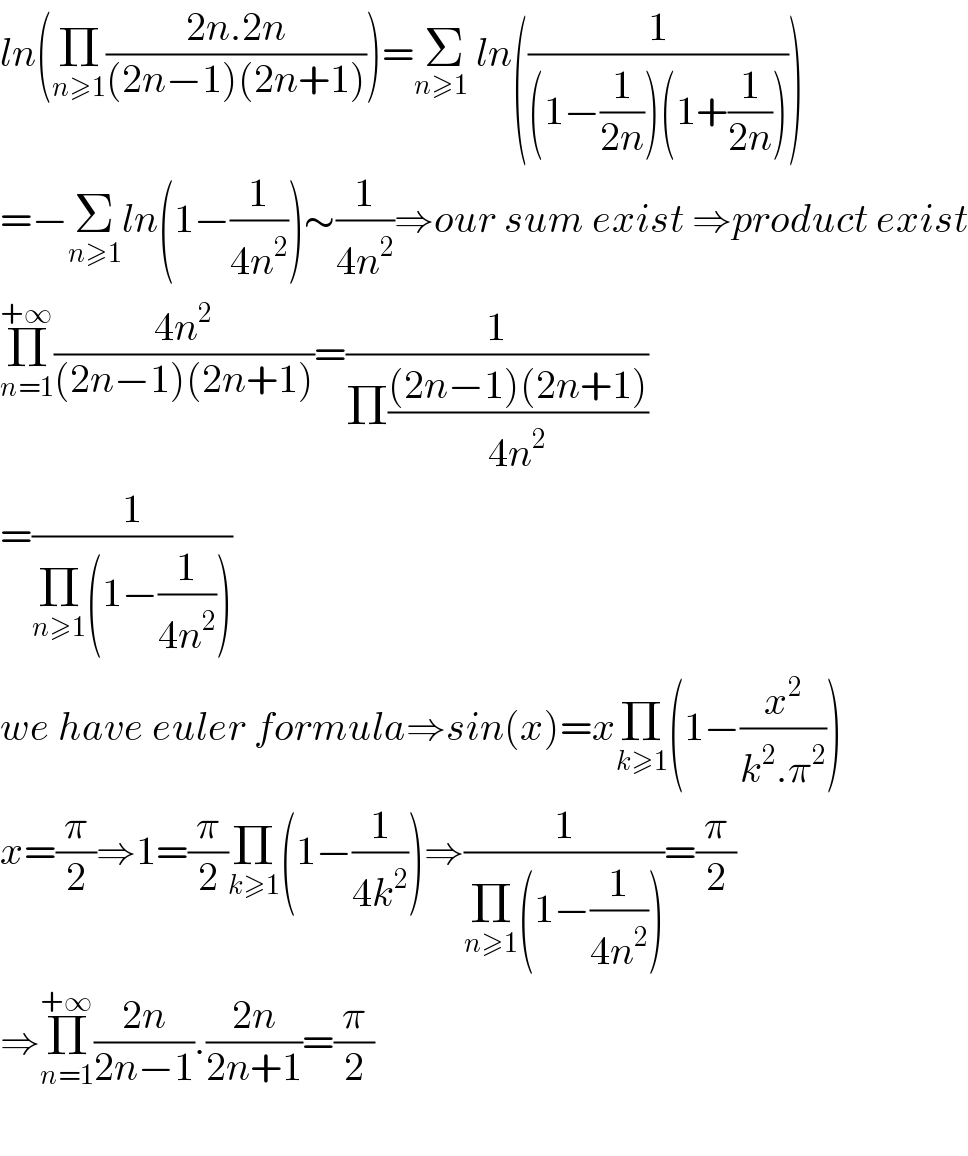
![Π_(n=1) ^∞ [((2n)/(2n−1)).((2n)/(2n+1))] =?](Q80792.png)
Answered by mind is power last updated on 06/Feb/20
Commented by jagoll last updated on 07/Feb/20

| ||
Question and Answers Forum | ||
Question Number 80792 by john santu last updated on 06/Feb/20 | ||
![Π_(n=1) ^∞ [((2n)/(2n−1)).((2n)/(2n+1))] =?](Q80792.png) | ||
Answered by mind is power last updated on 06/Feb/20 | ||
 | ||
| ||
Commented by jagoll last updated on 07/Feb/20 | ||
 | ||