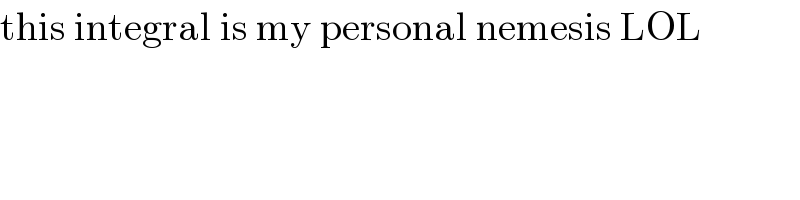Question and Answers Forum
Question Number 38451 by MJS last updated on 25/Jun/18
![new attempt to solve qu. 37630 ∫(dx/((√x)+(√(x+1))+(√(x+2))))= [t=x+1 → dx=dt] =∫(dt/((√(t−1))+(√t)+(√(t+1))))= [((to omit the roots)),(((√a)+(√b)+(√c) must be multiplied with)),(((−(√a)−(√b)+(√c))(−(√a)+(√b)−(√c))((√a)−(√b)−(√c)))),(((1/((√a)+(√b)+(√c)))=((a^(3/2) +b^(3/2) +c^(3/2) +2(√(abc))−((a+b)(√c)+(a+c)(√b)+(b+c)(√a)))/(a^2 +b^2 +c^2 −2(ab+ac+bc))))) ] =∫((t(√(t−1))+t(√t)+t(√(t+1))+2(√(t−1))−2(√(t+1))−2(√((t−1)t(t+1))))/(3t^2 −4))dt= =∫((t(√(t−1)))/(3t^2 −4))dt+∫((t(√t))/(3t^2 −4))dt+∫((t(√(t+1)))/(3t^2 −4))dt+2∫((√(t−1))/(3t^2 −4))dt−2∫((√(t+1))/(3t^2 −4))−2∫((√((t−1)t(t+1)))/(3t^2 −4))dt I think I can solve them all except the last one so please somebody try ∫((√((t−1)t(t+1)))/(3t^2 −4))dt=? I will do the others tomorrow](Q38451.png)
Commented by math khazana by abdo last updated on 26/Jun/18
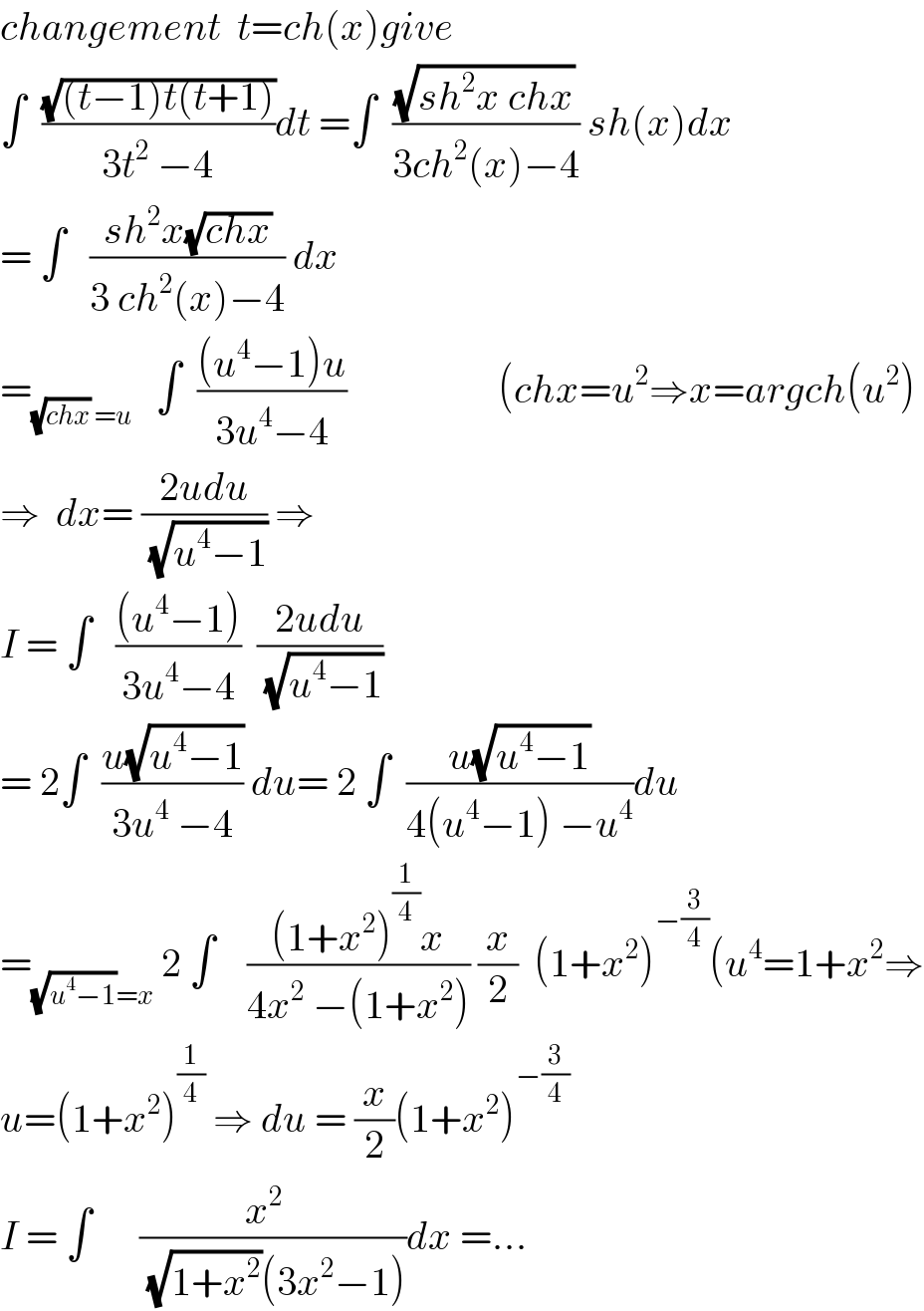
Answered by MJS last updated on 27/Jun/18
![∫((t(√(t−1)))/(3t^2 −4))dt= [u=(√(t−1)) → dt=2(√(t−1))du] =2∫((u^4 +u^2 )/(3u^4 +6u^2 −1))du=(2/3)∫(1−((3u^2 −1)/(3u^4 +6u^2 −1)))du= =(2/3)∫du+(2/3)∫(du/(3u^4 +6u^2 −1))−2∫(u^2 /(3u^4 +6u^2 −1))du= (2/3)∫du=(2/3)u=(2/3)(√(t−1)) (2/3)∫(du/(3u^4 +6u^2 −1))=(2/9)∫(du/(u^4 +2u^2 −(1/3)))= =(2/9)∫(du/((u^2 +((3−2(√3))/3))(u^2 +((3+2(√3))/3))))= =(2/9)∫(A/(u^2 +((3−2(√3))/3)))du+(2/9)∫(B/(u^2 +((3+2(√3))/3)))du= =((√3)/(18))∫(du/(u^2 +((3−2(√3))/3)))−((√3)/(18))∫(du/(u^2 +((3+2(√3))/3)))= [∫(dv/(v^2 −p^2 ))=(1/(2p))ln ((v−p)/(v+p)); ∫(dv/(v^2 +q^2 ))=(1/q)arctan (v/q)] =((√3)/(36))(√(3+2(√3)))ln∣((u−((√3)/3)(√(−3+2(√3))))/(u+((√3)/3)(√(−3+2(√3)))))∣−((√3)/(18))(√(−3+2(√3)))arctan (√(−3+2(√3)))u= =((√3)/(36))((√(3+2(√3)))ln∣(((√(t−1))−((√3)/3)(√(−3+2(√3))))/((√(t−1))+((√3)/3)(√(−3+2(√3)))))∣−2(√(−3+2(√3)))arctan (√((−3+2(√3))(t−1)))) −2∫(u^2 /(3u^4 +6u^2 −1))du=−(2/3)∫(u^2 /(u^4 +2u^2 −(1/3)))du= =−(2/3)∫(u^2 /((u^2 +((3−2(√3))/3))(u^2 +((3+2(√3))/3))))du= =−(2/3)∫(C/(u^2 +((3−2(√3))/3)))du−(2/3)∫(D/(u^2 +((3+2(√3))/3)))du= =−((2−(√3))/6)∫(du/(u^2 +((3−2(√3))/3)))−((2+(√3))/6)∫(du/(u^2 +((3+2(√3))/3)))= =−(1/(12))((√(−3+2(√3)))ln∣((u−((√3)/3)(√(−3+2(√3))))/(u+((√3)/3)(√(−3+2(√3)))))∣+2(√(3+2(√3)))arctan (√(−3+2(√3)))u)= =−(1/(12))((√(−3+2(√3)))ln∣(((√(t−1))−((√3)/3)(√(−3+2(√3))))/((√(t−1))+((√3)/3)(√(−3+2(√3)))))∣+2(√(3+2(√3)))arctan (√((−3+2(√3))(t−1)))) =(2/3)(√(t−1))+((√3)/(18))(√(−3+2(√3)))ln∣(((√(t−1))−((√3)/3)(√(−3+2(√3))))/((√(t−1))+((√3)/3)(√(−3+2(√3)))))∣+((√3)/9)(√(3+2(√3)))arctan (√((−3+2(√3))(t−1)))](Q38537.png)
Commented by MJS last updated on 27/Jun/18
![∫((t(√(t+1)))/(3t^2 −4))dt= [same procedure as above] =(2/3)(√(t+1))+((√3)/(18))(√(3+2(√3)))ln∣(((√(t+1))−((√3)/3)(√(3+2(√3))))/((√(t+1))+((√3)/3)(√(3+2(√3)))))∣−((√3)/9)(√(−3+2(√3)))arctan (√((3+2(√3))(t+1)))](Q38539.png)
Commented by MJS last updated on 27/Jun/18
![∫((t(√t))/(3t^2 −4))dt= [ditto] =(2/3)(√t)+((√(6(√3)))/(18))ln∣(((√t)−((√(6(√3)))/3))/((√t)+((√(6(√3)))/3)))∣−((√(6(√3)))/9)arctan ((√(2(√3)t))/2)](Q38541.png)
Commented by MJS last updated on 27/Jun/18
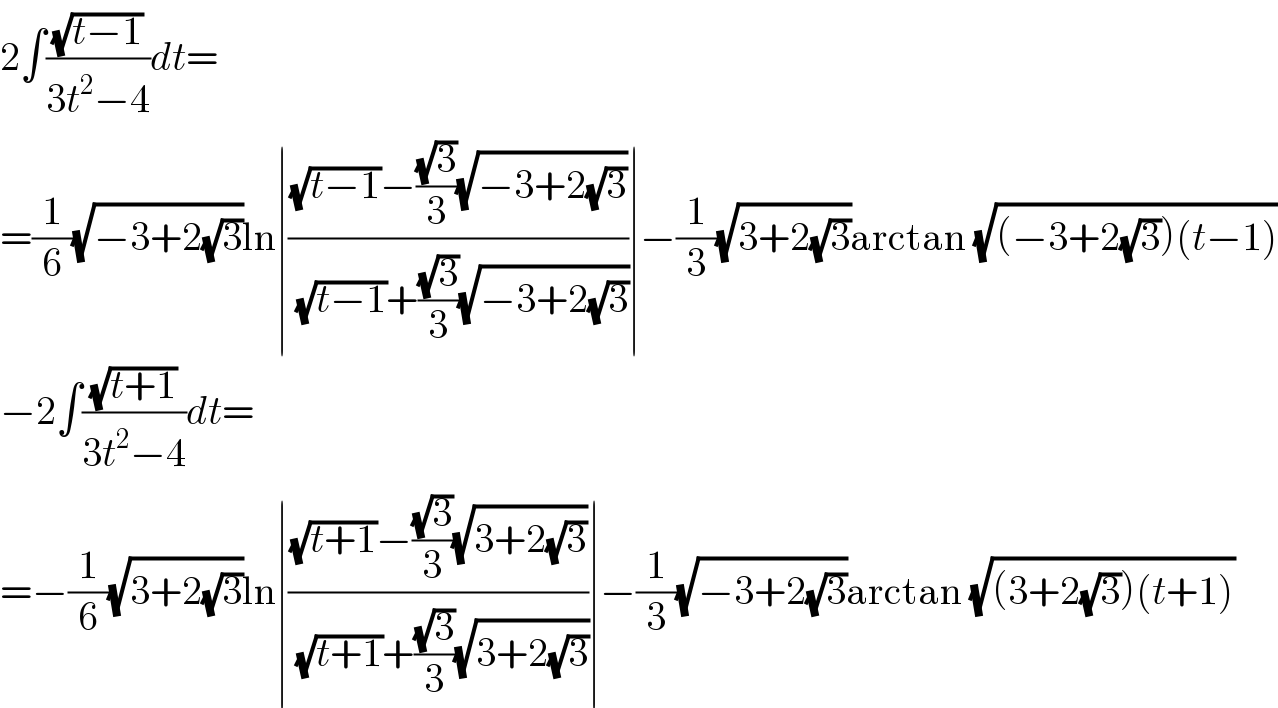
Commented by MJS last updated on 27/Jun/18
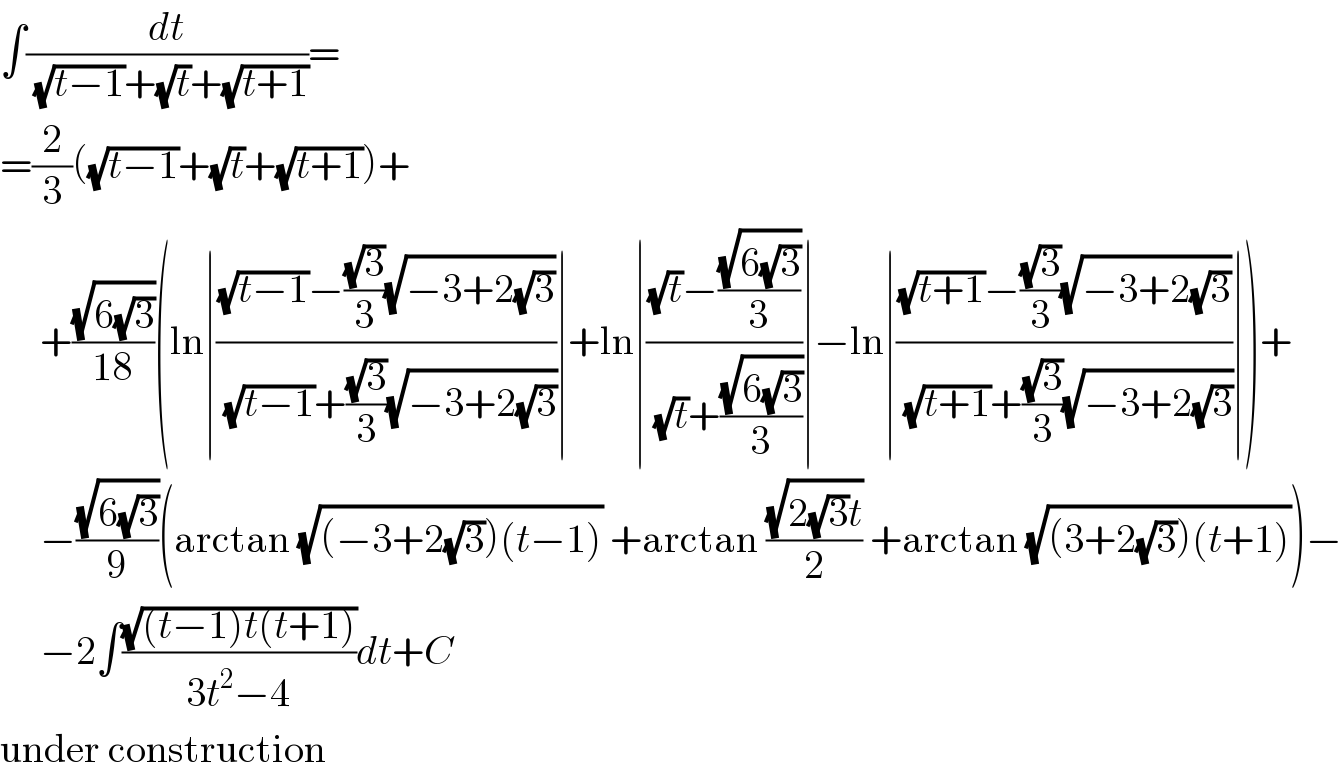
Commented by math khazana by abdo last updated on 27/Jun/18

Commented by MJS last updated on 27/Jun/18