
Question and Answers Forum
Question Number 114044 by Her_Majesty last updated on 16/Sep/20
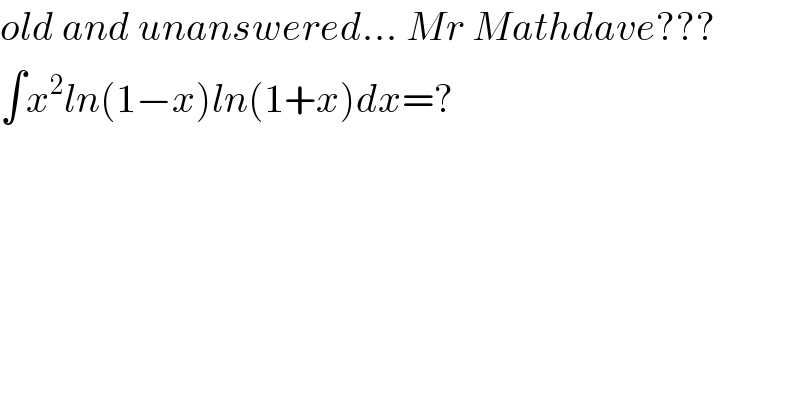
Answered by mathdave last updated on 17/Sep/20
![sokution put x=(2y−1) (wat i did here is logical) I=∫_0 ^1 (2y−1)^2 ln(2−2y)ln(2y)dy I=ln^2 (2)∫_0 ^1 (2y−1)^2 dy+ln2∫_0 ^1 (2y−1)^2 lnydy+ln2∫_0 ^1 (2y−1)^2 ln(1−y)dy+∫_0 ^1 (2y−1)^2 lnyln(1−y)dy let I_1 =ln^2 (2)∫_0 ^1 (2y−1)^2 dy=((ln^2 (2))/3).....(1) I_2 =ln2∫_0 ^1 (2y−1)^2 lnydy+ln2∫_0 ^1 (2y−1)^2 ln(1−y)dy I_2 =2ln2∫_0 ^1 (2y−1)^2 lnydy (∂/∂a)∣_(a=1) I_2 =2ln2∫_0 ^1 (2y−1)y^(a−1) dy (∂/∂a)∣_(a=1) I_2 =((2ln2•4)/((2+a))),I_2 =−((8ln2)/9).......(2) I_3 =∫_0 ^1 (2y−1)^2 ln(1−y)lnydy but (2y−1)^2 =4y^2 −4y+1 I_3 =4∫_0 ^1 y^2 ln(1−y)lnydy−4∫_0 ^1 yln(1−y)lnydy+∫_0 ^1 ln(1−y)lnydy I_3 =(∂/∂a)∣_(a=1) −4Σ_(n=1) ^∞ (1/n)∫_0 ^1 y^n .y^2 .y^(a−1) dy+(∂/∂a)∣_(a=1) Σ_(n=1) ^∞ (1/n)∫_0 ^1 y^n .y.y^(a−1) dy+(∂/∂a)∣_(a=1) Σ_(n=1) ^∞ (1/n)∫_0 ^1 y^n .y^(a−1) dy I_3 =(∂/∂a)∣_(a=1) [−4Σ_(n=1) ^∞ (1/(n(n+2+a)))+4Σ_(n=1) ^∞ (1/(n(n+1+a)))−Σ_(n=1) ^∞ (1/(n(n+a)))] I_3 =4Σ_(n=1) ^∞ (1/(n(n+3)^2 ))−4Σ_(n=1) ^∞ (1/(n(n+2)^2 ))+Σ_(n=1) ^∞ (1/(n(n+1)^2 )) I_3 =4[Σ_(n=1) ^∞ ((1/(9n))−(1/(9(n+3)))−(1/(3(n+3)^2 )))]−4[Σ_(n=1) ^∞ ((1/(4n))−(1/(4(n+2)))−(1/(2(n+2)^2 )))]+Σ_(n=1) ^∞ ((1/n)−(1/((n+1)^2 ))−(1/((n+1)))) I_3 =((71)/(27))−((4π^2 )/(18))+(π^2 /3)−4+2−(π^2 /6)=(((17)/(27))−(π^2 /(18))).....(3) but I=I_1 +I_2 +I_3 I=((ln^2 (2))/3)−((8ln2)/9)−(π^2 /(18))+((17)/(27)) ∵∫_0 ^1 x^2 ln(1−x)ln(1+x)dx=((ln^2 (2))/3)−((8ln2)/9)−(π^2 /(18))+((17)/(27)) mathdave (i try to compressed d work and skip some steps) any complain about the working is allow](Q114050.png)
Commented by mnjuly1970 last updated on 17/Sep/20
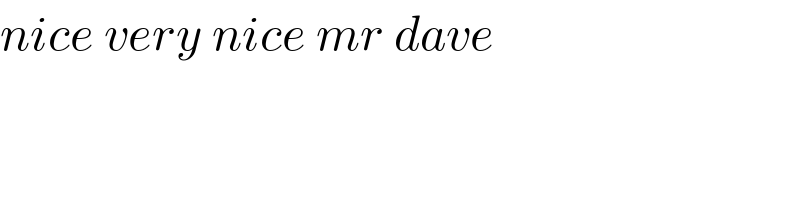
Commented by Tawa11 last updated on 06/Sep/21

| ||
Question and Answers Forum | ||
Question Number 114044 by Her_Majesty last updated on 16/Sep/20 | ||
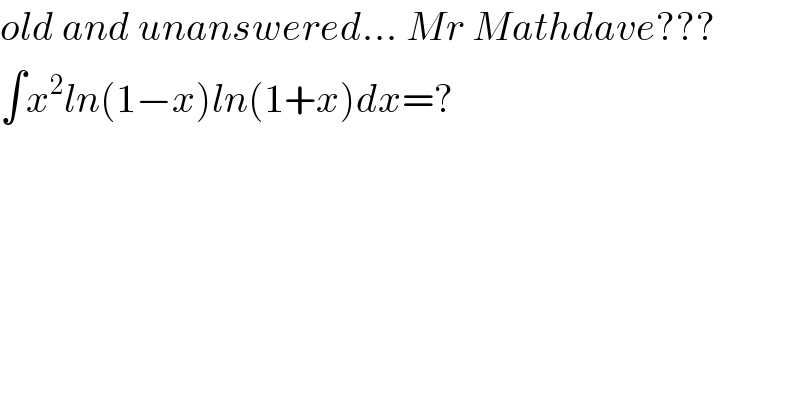 | ||
Answered by mathdave last updated on 17/Sep/20 | ||
![sokution put x=(2y−1) (wat i did here is logical) I=∫_0 ^1 (2y−1)^2 ln(2−2y)ln(2y)dy I=ln^2 (2)∫_0 ^1 (2y−1)^2 dy+ln2∫_0 ^1 (2y−1)^2 lnydy+ln2∫_0 ^1 (2y−1)^2 ln(1−y)dy+∫_0 ^1 (2y−1)^2 lnyln(1−y)dy let I_1 =ln^2 (2)∫_0 ^1 (2y−1)^2 dy=((ln^2 (2))/3).....(1) I_2 =ln2∫_0 ^1 (2y−1)^2 lnydy+ln2∫_0 ^1 (2y−1)^2 ln(1−y)dy I_2 =2ln2∫_0 ^1 (2y−1)^2 lnydy (∂/∂a)∣_(a=1) I_2 =2ln2∫_0 ^1 (2y−1)y^(a−1) dy (∂/∂a)∣_(a=1) I_2 =((2ln2•4)/((2+a))),I_2 =−((8ln2)/9).......(2) I_3 =∫_0 ^1 (2y−1)^2 ln(1−y)lnydy but (2y−1)^2 =4y^2 −4y+1 I_3 =4∫_0 ^1 y^2 ln(1−y)lnydy−4∫_0 ^1 yln(1−y)lnydy+∫_0 ^1 ln(1−y)lnydy I_3 =(∂/∂a)∣_(a=1) −4Σ_(n=1) ^∞ (1/n)∫_0 ^1 y^n .y^2 .y^(a−1) dy+(∂/∂a)∣_(a=1) Σ_(n=1) ^∞ (1/n)∫_0 ^1 y^n .y.y^(a−1) dy+(∂/∂a)∣_(a=1) Σ_(n=1) ^∞ (1/n)∫_0 ^1 y^n .y^(a−1) dy I_3 =(∂/∂a)∣_(a=1) [−4Σ_(n=1) ^∞ (1/(n(n+2+a)))+4Σ_(n=1) ^∞ (1/(n(n+1+a)))−Σ_(n=1) ^∞ (1/(n(n+a)))] I_3 =4Σ_(n=1) ^∞ (1/(n(n+3)^2 ))−4Σ_(n=1) ^∞ (1/(n(n+2)^2 ))+Σ_(n=1) ^∞ (1/(n(n+1)^2 )) I_3 =4[Σ_(n=1) ^∞ ((1/(9n))−(1/(9(n+3)))−(1/(3(n+3)^2 )))]−4[Σ_(n=1) ^∞ ((1/(4n))−(1/(4(n+2)))−(1/(2(n+2)^2 )))]+Σ_(n=1) ^∞ ((1/n)−(1/((n+1)^2 ))−(1/((n+1)))) I_3 =((71)/(27))−((4π^2 )/(18))+(π^2 /3)−4+2−(π^2 /6)=(((17)/(27))−(π^2 /(18))).....(3) but I=I_1 +I_2 +I_3 I=((ln^2 (2))/3)−((8ln2)/9)−(π^2 /(18))+((17)/(27)) ∵∫_0 ^1 x^2 ln(1−x)ln(1+x)dx=((ln^2 (2))/3)−((8ln2)/9)−(π^2 /(18))+((17)/(27)) mathdave (i try to compressed d work and skip some steps) any complain about the working is allow](Q114050.png) | ||
| ||
Commented by mnjuly1970 last updated on 17/Sep/20 | ||
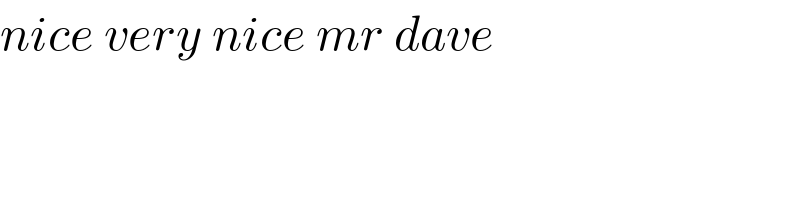 | ||
Commented by Tawa11 last updated on 06/Sep/21 | ||
 | ||