prove that Ω =∫_(−∞) ^( +∞) (( cos (x))/((2+ 2x +x^( 2) )^( 2) )) dx = (π/e) cos(1)


Question and Answers Forum

All Questions Topic List
Integration Questions
Previous in All Question Next in All Question
Previous in Integration Next in Integration
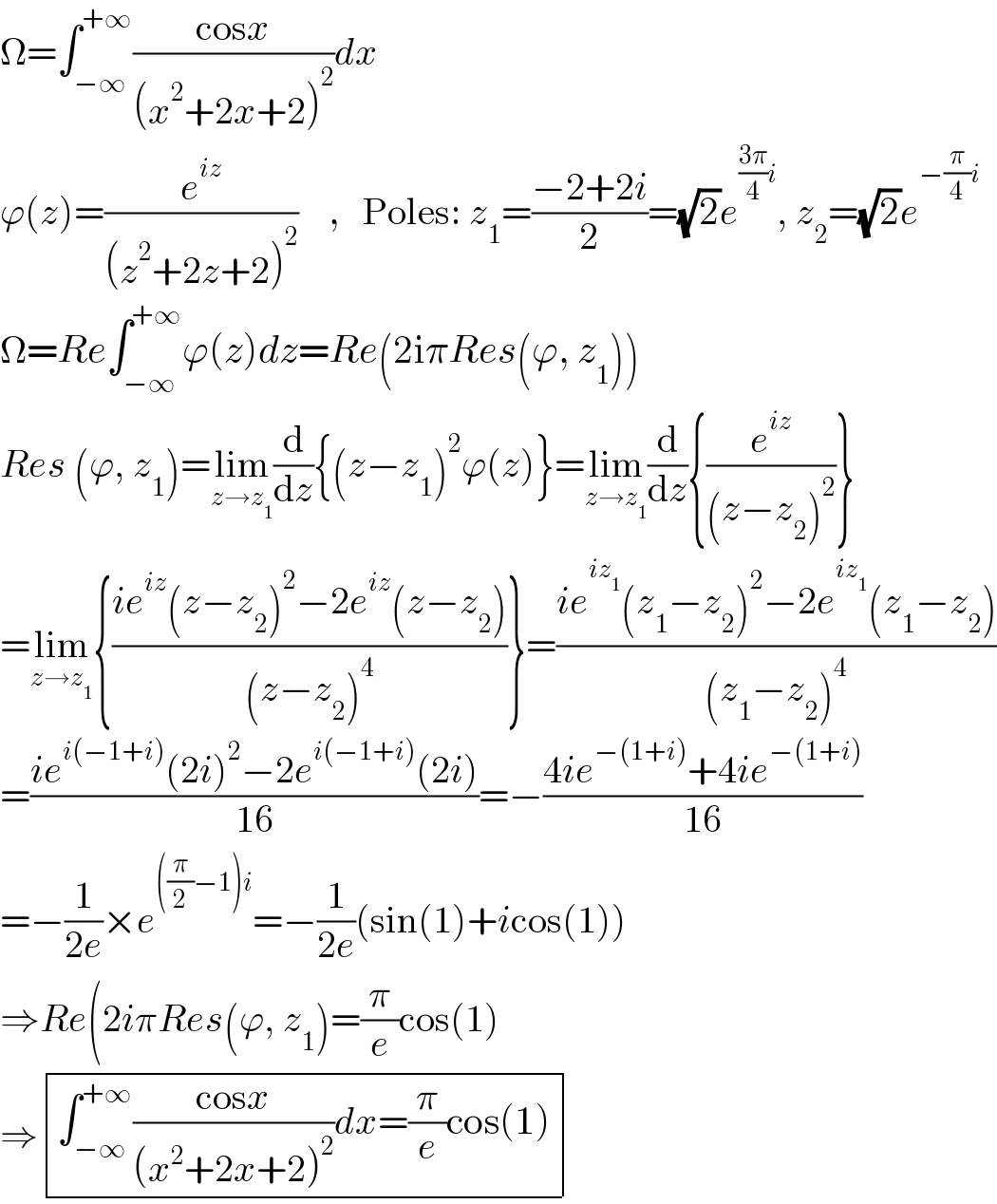
Question Number 162073 by mnjuly1970 last updated on 26/Dec/21

$p r o v e t h a t$ $Ω = \int_{- \infty}^{+ \infty} \frac{c o s (x)}{{(2 + 2 x + x^{2})}^{2}} d x = \frac{π}{e} c o s (1)$
	Answered by mindispower last updated on 26/Dec/21

	$Ω = \int_{- \infty}^{\infty} \frac{c o s (x)}{{(1 + {(1 + x)}^{2})}^{2}} d x$ $1 + x = t$ $= \int_{- \infty}^{\infty} \frac{c o s (t - 1)}{{(1 + t^{2})}^{2}} d t = \int_{- \infty}^{\infty} \frac{c o s (t) c o s (1)}{{(1 + t^{2})}^{2}} d t + s i n (1) \int_{- \infty}^{\infty} \frac{s i n (t)}{{(1 + t^{2})}^{2}} {d t}_{= 0}$ $= c o s (1) \int_{- \infty}^{\infty} \frac{c o s (t) d t}{{(1 + t^{2})}^{2}}$ $f (a) = \int_{- \infty}^{\infty} \frac{c o s (t)}{a^{2} + t^{2}} = R e \int_{- \infty}^{\infty} \frac{e^{i t}}{a^{2} + t^{2}} = R e (2 i π . e^{- a} . \frac{1}{2 i a})$ $= \frac{π}{a} e^{- a} = f (a)$ $f^{'} (a) = \int_{- \infty}^{\infty} \frac{- 2 a c o s (t)}{{(a^{2} + t^{2})}^{2}} d t$ $f^{'} (1) = - 2 \int_{- \infty}^{\infty} \frac{c o s (t) d t}{{(1 + t^{2})}^{2}}$ $f^{'} (a) = (- \frac{π}{a^{2}} e^{- a} - \frac{π}{a} e^{- a})$ $\int_{- \infty}^{\infty} \frac{c o s (t) d t}{{(1 + t^{2})}^{2}} = \frac{1}{2} . \frac{2 π}{e} = \frac{π}{e}$ $\int_{- \infty}^{\infty} \frac{c o s t)}{{(2 + 2 t + t^{2})}^{2}} d t = c o s (1) \int \frac{c o s (x)}{{(1 + x^{2})}^{2}} d x = c o s (1) \frac{π}{e}$
	Commented by Tawa11 last updated on 26/Dec/21

	$Great sir$
	Commented by mnjuly1970 last updated on 26/Dec/21

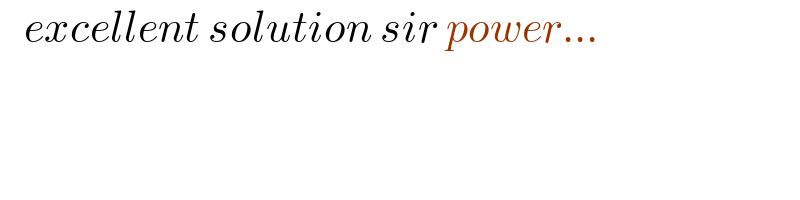
	$e x c e l l e n t s o l u t i o n s i r p o w e r . . .$
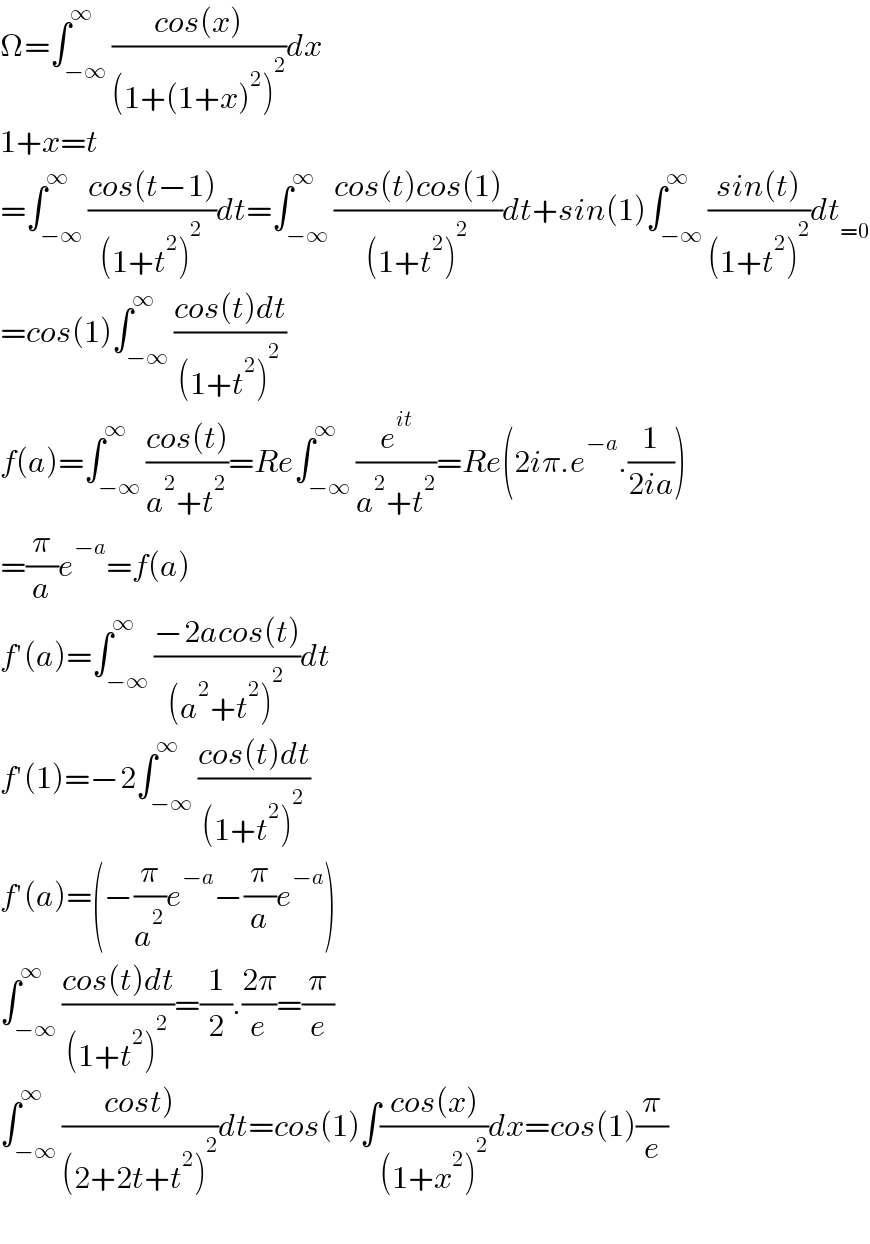
	Answered by Mathspace last updated on 26/Dec/21
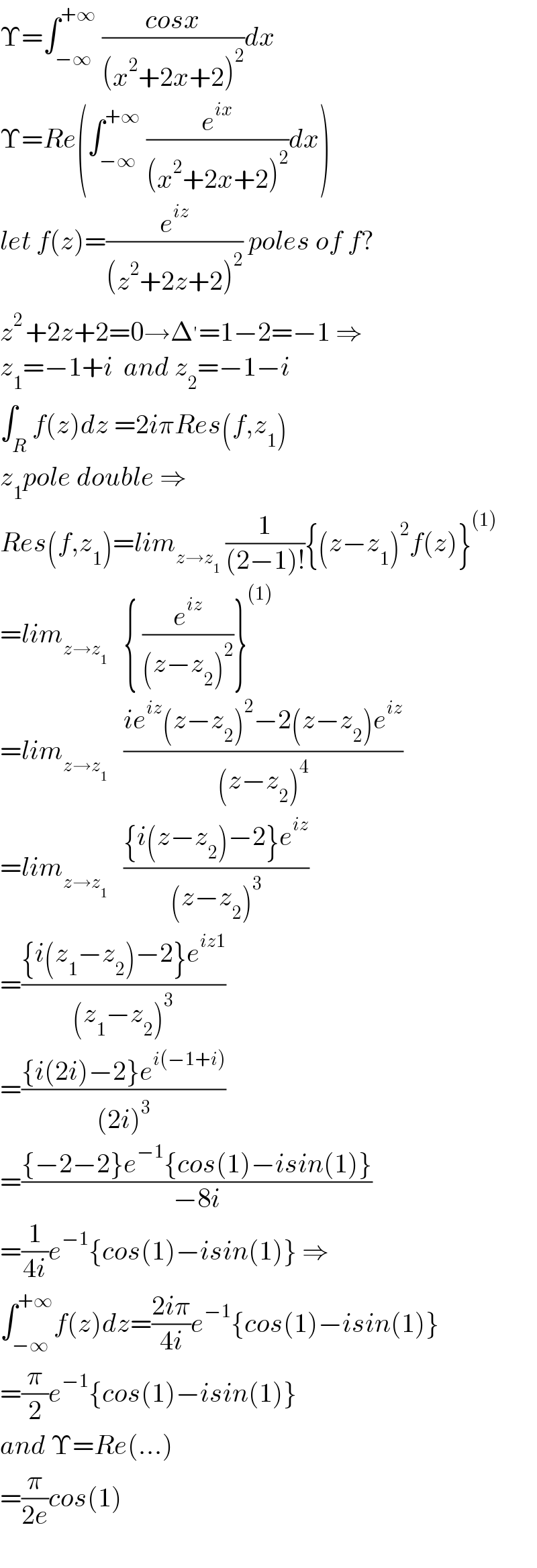
	$Υ=∫_(−∞) ^(+∞) ((cosx)/((x^2 +2x+2)^2 ))dx Υ=Re(∫_(−∞) ^(+∞) (e^(ix) /((x^2 +2x+2)^2 ))dx) let f(z)=(e^(iz) /((z^2 +2z+2)^2 )) poles of f? z^(2 ) +2z+2=0→Δ^′ =1−2=−1 ⇒ z_1 =−1+i and z_2 =−1−i ∫_R f(z)dz =2iπRes(f,z_1 ) z_1 pole double ⇒ Res(f,z_1 )=lim_(z→z_1 ) (1/((2−1)!)){(z−z_1 )^2 f(z)}^((1)) =lim_(z→z_1 ) { (e^(iz) /((z−z_2 )^2 ))}^((1)) =lim_(z→z_1 ) ((ie^(iz) (z−z_2 )^2 −2(z−z_2 )e^(iz) )/((z−z_2 )^4 )) =lim_(z→z_1 ) (({i(z−z_2 )−2}e^(iz) )/((z−z_2 )^3 )) =(({i(z_1 −z_2 )−2}e^(iz1) )/((z_1 −z_2 )^3 )) =(({i(2i)−2}e^(i(−1+i)) )/((2i)^3 )) =(({−2−2}e^(−1) {cos(1)−isin(1)})/(−8i)) =(1/(4i))e^(−1) {cos(1)−isin(1)} ⇒ ∫_(−∞) ^(+∞) f(z)dz=((2iπ)/(4i))e^(−1) {cos(1)−isin(1)} =(π/2)e^(−1) {cos(1)−isin(1)} and Υ=Re(...) =(π/(2e))cos(1)$
	$Υ = \int_{- \infty}^{+ \infty} \frac{c o s x}{{(x^{2} + 2 x + 2)}^{2}} d x$ $Υ = R e (\int_{- \infty}^{+ \infty} \frac{e^{i x}}{{(x^{2} + 2 x + 2)}^{2}} d x)$ $l e t f (z) = \frac{e^{i z}}{{(z^{2} + 2 z + 2)}^{2}} p o l e s o f f ?$ $z^{2} + 2 z + 2 = 0 \to Δ^{^{'}} = 1 - 2 = - 1 \Rightarrow$ $z_{1} = - 1 + i a n d z_{2} = - 1 - i$ $\int_{R} f (z) d z = 2 i π R e s (f, z_{1})$ $z_{1} p o l e d o u b l e \Rightarrow$ $R e s (f, z_{1}) = {l i m}_{z \to z_{1}} \frac{1}{(2 - 1)!} {{(z - z_{1})}^{2} f (z)}^{(1)}$ $= {l i m}_{z \to z_{1}} {\frac{e^{i z}}{{(z - z_{2})}^{2}}}^{(1)}$ $= {l i m}_{z \to z_{1}} \frac{{i e}^{i z} {(z - z_{2})}^{2} - 2 (z - z_{2}) e^{i z}}{{(z - z_{2})}^{4}}$ $= {l i m}_{z \to z_{1}} \frac{{i (z - z_{2}) - 2} e^{i z}}{{(z - z_{2})}^{3}}$ $= \frac{{i (z_{1} - z_{2}) - 2} e^{i z 1}}{{(z_{1} - z_{2})}^{3}}$ $= \frac{{i (2 i) - 2} e^{i (- 1 + i)}}{{(2 i)}^{3}}$ $= \frac{{- 2 - 2} e^{- 1} {c o s (1) - i s i n (1)}}{- 8 i}$ $= \frac{1}{4 i} e^{- 1} {c o s (1) - i s i n (1)} \Rightarrow$ $\int_{- \infty}^{+ \infty} f (z) d z = \frac{2 i π}{4 i} e^{- 1} {c o s (1) - i s i n (1)}$ $= \frac{π}{2} e^{- 1} {c o s (1) - i s i n (1)}$ $a n d Υ = R e (. . .)$ $= \frac{π}{2 e} c o s (1)$
	Commented by mnjuly1970 last updated on 26/Dec/21

	$t h a n k s a l o t m a s t e r$
	Answered by Ar Brandon last updated on 24/Mar/22
	$Ω=∫_(−∞) ^(+∞) ((cosx)/((x^2 +2x+2)^2 ))dx ϕ(z)=(e^(iz) /((z^2 +2z+2)^2 )) , Poles: z_1 =((−2+2i)/2)=(√2)e^(((3π)/4)i) , z_2 =(√2)e^(−(π/4)i) Ω=Re∫_(−∞) ^(+∞) ϕ(z)dz=Re(2iπRes(ϕ, z_1 )) Res (ϕ, z_1 )=lim_(z→z_1 ) (d/dz){(z−z_1 )^2 ϕ(z)}=lim_(z→z_1 ) (d/dz){(e^(iz) /((z−z_2 )^2 ))} =lim_(z→z_1 ) {((ie^(iz) (z−z_2 )^2 −2e^(iz) (z−z_2 ))/((z−z_2 )^4 ))}=((ie^(iz_1 ) (z_1 −z_2 )^2 −2e^(iz_1 ) (z_1 −z_2 ))/((z_1 −z_2 )^4 )) =((ie^(i(−1+i)) (2i)^2 −2e^(i(−1+i)) (2i))/(16))=−((4ie^(−(1+i)) +4ie^(−(1+i)) )/(16)) =−(1/(2e))×e^(((π/2)−1)i) =−(1/(2e))(sin(1)+icos(1)) ⇒Re(2iπRes(ϕ, z_1 )=(π/e)cos(1) ⇒ determinant (((∫_(−∞) ^(+∞) ((cosx)/((x^2 +2x+2)^2 ))dx=(π/e)cos(1))))$
	$Ω = \int_{- \infty}^{+ \infty} \frac{\cos x}{{(x^{2} + 2 x + 2)}^{2}} d x$ $φ (z) = \frac{e^{i z}}{{(z^{2} + 2 z + 2)}^{2}}, Poles : z_{1} = \frac{- 2 + 2 i}{2} = \sqrt{2} e^{\frac{3 π}{4} i}, z_{2} = \sqrt{2} e^{- \frac{π}{4} i}$ $Ω = R e \int_{- \infty}^{+ \infty} φ (z) d z = R e (2 i π R e s (φ, z_{1}))$ $R e s (φ, z_{1}) = \lim_{z \to z_{1}} \frac{d}{d z} {{(z - z_{1})}^{2} φ (z)} = \lim_{z \to z_{1}} \frac{d}{d z} {\frac{e^{i z}}{{(z - z_{2})}^{2}}}$ $= \lim_{z \to z_{1}} {\frac{{i e}^{i z} {(z - z_{2})}^{2} - 2 e^{i z} (z - z_{2})}{{(z - z_{2})}^{4}}} = \frac{{i e}^{{i z}_{1}} {(z_{1} - z_{2})}^{2} - 2 e^{{i z}_{1}} (z_{1} - z_{2})}{{(z_{1} - z_{2})}^{4}}$ $= \frac{{i e}^{i (- 1 + i)} {(2 i)}^{2} - 2 e^{i (- 1 + i)} (2 i)}{16} = - \frac{4 {i e}^{- (1 + i)} + 4 {i e}^{- (1 + i)}}{16}$ $= - \frac{1}{2 e} \times e^{(\frac{π}{2} - 1) i} = - \frac{1}{2 e} (\sin (1) + i \cos (1))$ $\Rightarrow R e (2 i π R e s (φ, z_{1}) = \frac{π}{e} \cos (1)$ $\Rightarrow \begin{matrix} \int_{- \infty}^{+ \infty} \frac{\cos x}{{(x^{2} + 2 x + 2)}^{2}} d x = \frac{π}{e} \cos (1) \end{matrix}$
Terms of Service
Privacy Policy
Contact: info@tinkutara.com

Question and Answers Forum