⋓si⋒g ChineseRemainderTheorm ∂etermine polynomial p(x) such that p(x)≡8(mod x+1) p(x)≡−24(mod x+3) p(x)≡6(mod x) p(x)≡0(mod x+2)


Question and Answers Forum

All Questions Topic List
Others Questions
Previous in All Question Next in All Question
Previous in Others Next in Others
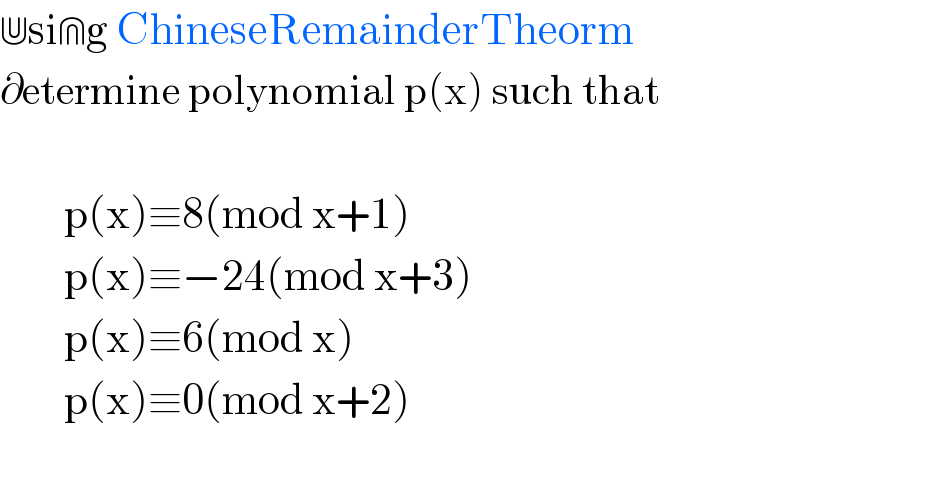
Question Number 67697 by Rasheed.Sindhi last updated on 30/Aug/19

$⋓ si ⋒ g ChineseRemainderTheorm$ $\partial etermine polynomial p (x) such that$ $p (x) \equiv 8 (\mod x + 1)$ $p (x) \equiv - 24 (\mod x + 3)$ $p (x) \equiv 6 (\mod x)$ $p (x) \equiv 0 (\mod x + 2)$
	Answered by Rasheed.Sindhi last updated on 01/Sep/19
	${ ((p(x)≡8(mod x+1))),((p(x)≡−24(mod x+3))),((p(x)≡6(mod x))),((p(x)≡0(mod x+2))) :} Let m_1 =x+1,m_2 =x+3,m_3 =x,m_4 =x+2 Note that (m_i ,m_j )=1 for ∀ i≠j (pairwise coprime) Let M=m_1 m_2 m_3 m_4 =(x+1)(x+3)(x)(x+2) M_1 =(M/m_1 )=m_2 m_3 m_4 =(x+3)(x)(x+2) M_2 =(M/m_2 )=m_1 m_3 m_4 =(x+1)(x)(x+2) M_3 =(M/m_3 )=m_1 m_2 m_4 =(x+1)(x+3)(x+2) M_4 =(M/m_4 )=m_1 m_2 m_3 =(x+1)(x+3)(x) Note that (M_i ,m_i )=1 ∴ M_i y≡1(mod m_i ) (x+3)(x)(x+2)H_1 (x)≡1(mod x+1) (x^3 +5x^2 +6x)H_1 (x)≡1(mod x+1) Let H_1 (x)=h_1 (a constant) (((−1)),h_1 ,( 5h_1 ),( 6h_1 ),( −1)),(,,(−h_1 ),(−4h_1 ),( −2h_1 )),(,h_1 ,( 4h_1 ),( 2h_1 ),( −2h_1 −1)) ) −2h_1 −1=0⇒h_1 =−1/2 (x+1)(x)(x+2)H_2 (x)≡1(mod x+3) (x^3 +3x^2 +2x)H_2 (x)≡1(mod x+3) If H_2 (x)=h_2 (constant) h_2 =−1/6 (By similar process) (x+1)(x+3)(x+2)H_3 (x)≡1(mod x) (x^3 +6x^2 +11x+6)H_3 (x)≡1(mod x) If H_3 (x)=h_3 (constant) (((0)),h_3 ,(6h_3 ),(11h_3 ),(6h_3 −1)),(,,0,0,0),(,h_3 ,(6h_3 ),(11h_3 ),(6h_3 −1)) ) 6h_3 −1=0⇒h_3 =1/6 (x+1)(x+3)(x)H_4 (x)≡1(mod x+2) (x^3 +4x^2 +3x)H_4 (x)≡1(mod x+2) If H_4 (x)=h_4 (((−2)),h_4 ,( 4h_4 ),( 3h_4 ),(−1)),(,,(−2h4),(−4h_4 ),( 2h_4 )),(,h_4 ,( 2h_4 ),(−h_4 ),(2h_4 −1)) ) 2h_4 −1=0⇒h_4 =1/2 x=8(x^3 +5x^2 +6x)(−1/2) +(−24)(x^3 +3x^2 +2x)(−1/6) +6(x^3 +6x^2 +11x+6)(1/6) +(0)(x^3 +4x^2 +3x)(1/2) x=−4x^3 −20x^2 −24x +4x^3 +12x^2 + 8x + x^3 + 6x^2 +11x+6 x=x^3 −2x^2 −5x+6$
	${\begin{cases} p (x) \equiv 8 (\mod x + 1) \\ p (x) \equiv - 24 (\mod x + 3) \\ p (x) \equiv 6 (\mod x) \\ p (x) \equiv 0 (\mod x + 2) \end{cases}$ $Let m_{1} = x + 1, m_{2} = x + 3, m_{3} = x, m_{4} = x + 2$ $Note that (m_{i}, m_{j}) = 1 for \forall i \neq j$ $(pairwise coprime)$ $Let M = m_{1} m_{2} m_{3} m_{4} = (x + 1) (x + 3) (x) (x + 2)$ $M_{1} = \frac{M}{m_{1}} = m_{2} m_{3} m_{4} = (x + 3) (x) (x + 2)$ $M_{2} = \frac{M}{m_{2}} = m_{1} m_{3} m_{4} = (x + 1) (x) (x + 2)$ $M_{3} = \frac{M}{m_{3}} = m_{1} m_{2} m_{4} = (x + 1) (x + 3) (x + 2)$ $M_{4} = \frac{M}{m_{4}} = m_{1} m_{2} m_{3} = (x + 1) (x + 3) (x)$ $Note that (M_{i}, m_{i}) = 1$ $∴ M_{i} y \equiv 1 (\mod m_{i})$ $(x + 3) (x) (x + 2) H_{1} (x) \equiv 1 (\mod x + 1)$ $(x^{3} + {5 x}^{2} + 6 x) H_{1} (x) \equiv 1 (\mod x + 1)$ $Let H_{1} (x) = h_{1} (a constant)$ $(\begin{matrix} - 1) & h_{1} & {5 h}_{1} & {6 h}_{1} & - 1 \\ - h_{1} & - {4 h}_{1} & - {2 h}_{1} \\ h_{1} & {4 h}_{1} & {2 h}_{1} & - {2 h}_{1} - 1 \end{matrix})$ $- {2 h}_{1} - 1 = 0 \Rightarrow h_{1} = - 1 / 2$ $(x + 1) (x) (x + 2) H_{2} (x) \equiv 1 (\mod x + 3)$ $(x^{3} + {3 x}^{2} + 2 x) H_{2} (x) \equiv 1 (\mod x + 3)$ $If H_{2} (x) = h_{2} (constant)$ $h_{2} = - 1 / 6 (By similar process)$ $(x + 1) (x + 3) (x + 2) H_{3} (x) \equiv 1 (\mod x)$ $(x^{3} + {6 x}^{2} + 11 x + 6) H_{3} (x) \equiv 1 (\mod x)$ $If H_{3} (x) = h_{3} (constant)$ $(\begin{matrix} 0) & h_{3} & {6 h}_{3} & {11 h}_{3} & {6 h}_{3} - 1 \\ 0 & 0 & 0 \\ h_{3} & {6 h}_{3} & {11 h}_{3} & {6 h}_{3} - 1 \end{matrix})$ ${6 h}_{3} - 1 = 0 \Rightarrow h_{3} = 1 / 6$ $(x + 1) (x + 3) (x) H_{4} (x) \equiv 1 (\mod x + 2)$ $(x^{3} + {4 x}^{2} + 3 x) H_{4} (x) \equiv 1 (\mod x + 2)$ $If H_{4} (x) = h_{4}$ $(\begin{matrix} - 2) & h_{4} & {4 h}_{4} & {3 h}_{4} & - 1 \\ - 2 h 4 & - {4 h}_{4} & {2 h}_{4} \\ h_{4} & {2 h}_{4} & - h_{4} & {2 h}_{4} - 1 \end{matrix})$ ${2 h}_{4} - 1 = 0 \Rightarrow h_{4} = 1 / 2$ $x = 8 (x^{3} + {5 x}^{2} + 6 x) (- 1 / 2)$ $+ (- 24) (x^{3} + {3 x}^{2} + 2 x) (- 1 / 6)$ $+ 6 (x^{3} + {6 x}^{2} + 11 x + 6) (1 / 6)$ $+ (0) (x^{3} + {4 x}^{2} + 3 x) (1 / 2)$ $x = - {4 x}^{3} - {20 x}^{2} - 24 x$ $+ {4 x}^{3} + {12 x}^{2} + 8 x$ $+ x^{3} + {6 x}^{2} + 11 x + 6$ $x = x^{3} - {2 x}^{2} - 5 x + 6$
	Commented by Prithwish sen last updated on 02/Sep/19

	$excelent .$
	Commented by mr W last updated on 03/Sep/19

	$n i c e c r e a t i o n!$
	Commented by Rasheed.Sindhi last updated on 04/Sep/19

	$Thank you both sirs!$
Terms of Service
Privacy Policy
Contact: info@tinkutara.com

Question and Answers Forum