Question and Answers Forum

All Questions      Topic List

Geometry Questions

Previous in All Question      Next in All Question      

Previous in Geometry      Next in Geometry      

Question Number 11781 by tawa last updated on 31/Mar/17

$$\sin \left(2\theta \right)+7\cos \left(2\theta \right)=6$$$$\mathrm{find}\theta$$

Answered by sma3l2996 last updated on 31/Mar/17

$$2sin\left(\theta \right)cos\left(\theta \right)+7{cos}^2\left(\theta \right)-7{sin}^2\left(\theta \right)=6$$$$2sin\left(\theta \right)cos\left(\theta \right)+14{cos}^2\left(\theta \right)-7=6$$$$2sin\left(\theta \right)cos\left(\theta \right)=13-14{cos}^2\left(\theta \right)$$$$4(1-{cos}^2\left(\theta \right)){cos}^2\left(\theta \right)={13}^2+{14}^2{cos}^4\left(\theta \right)-2\times 13\times 14{cos}^2\left(\theta \right)$$$$4{cos}^2\left(\theta \right)-4{cos}^4\left(\theta \right)=169+196{cos}^4\left(\theta \right)-364{cos}^2\left(\theta \right)$$$$200{cos}^4\left(\theta \right)-368{cos}^2\left(\theta \right)+169=0$$$$200{cos}^4\left(\theta \right)-\frac{2\times 184\times 10\sqrt{2}}{10\sqrt{2}}{cos}^2\left(\theta \right)+{\left(\frac{184}{10\sqrt{2}}\right)}^2={\left(\frac{92}{5\sqrt{2}}\right)}^2-169$$$${(10\sqrt{2}{cos}^2\left(\theta \right)-\frac{92}{5\sqrt{2}})}^2=\frac{4232}{25}-169$$$$10\sqrt{2}{cos}^2\left(\theta \right)-\frac{92}{5\sqrt{2}}=\underset{-}{+}\frac{\sqrt{3387}}{5}$$$${cos}^2\left(\theta \right)=(\underset{-}{+}\frac{\sqrt{3387}}{5}+\frac{92}{5\sqrt{2}})\frac{1}{10\sqrt{2}}=\underset{-}{+}\frac{\sqrt{3387}}{50\sqrt{2}}+\frac{23}{25}$$$$cos\left(\theta \right)=\sqrt{\frac{\sqrt{3387}}{50\sqrt{2}}+\frac{23}{25}}$$$$\theta =acos\left(\sqrt{\frac{\sqrt{3387}}{50\sqrt{2}}+\frac{23}{25}}\right)+2k\pi$$

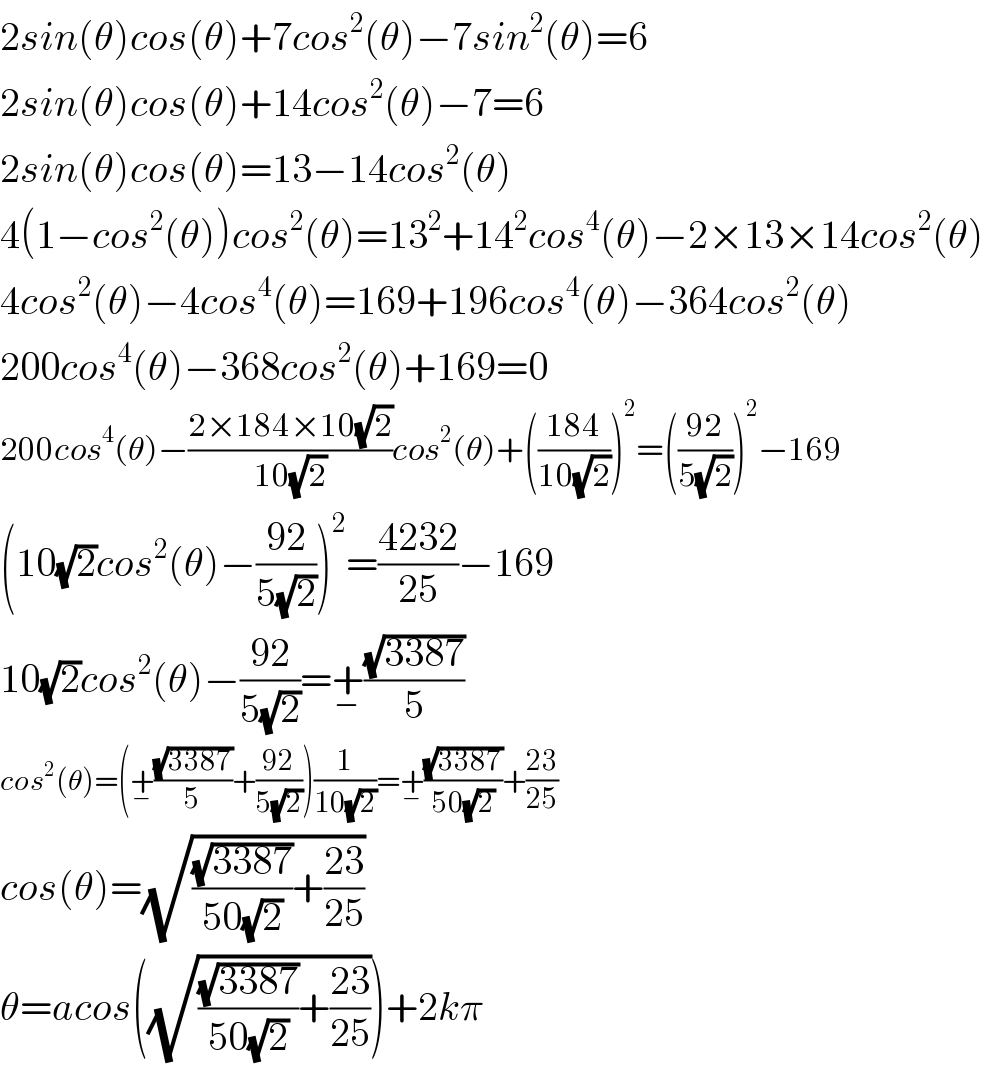
Commented by tawa last updated on 31/Mar/17

$$\mathrm{God}\mathrm{bless}\mathrm{you}\mathrm{sir}$$

Answered by sandy_suhendra last updated on 31/Mar/17

$$\sin 2\theta +7\cos 2\theta =\mathrm{k}\cos (2\theta -\alpha )$$$$=\mathrm{k}\cos 2\theta \cos \alpha +\mathrm{k}\sin 2\theta \sin \alpha$$$$\mathrm{thus}$$$$\mathrm{k}\cos \alpha =7\mathrm{and}\mathrm{k}\sin \alpha =1$$$${\left(\mathrm{k}\cos \alpha \right)}^2+{\left(\mathrm{k}\sin \alpha \right)}^2=7^2+1^2$$$${\mathrm{k}}^2({\sin }^2\alpha +{\cos }^2\alpha )=50$$$$\mathrm{k}=\sqrt{50}=5\sqrt{2}$$$$$$$$\frac{\mathrm{k}\sin \alpha }{\mathrm{k}\cos \alpha }=\tan \alpha \Rightarrow \tan \alpha =\frac{1}{7}\Rightarrow \alpha =8.13°$$$$5\sqrt{2}\cos (2\theta -8.13°)=6$$$$\cos (2\theta -8.13°)=\frac{6}{5\sqrt{2}}$$$$\left(1\right)2\theta -8.13°=31.95°+\mathrm{p}.360°$$$$2\theta =40.08°+\mathrm{p}.360°$$$$\theta =20.04+\mathrm{p}.180°\Rightarrow \mathrm{p}=0,1,2,...$$$$\left(2\right)2\theta -8.13°=-31.95°+\mathrm{p}.360°$$$$2\theta =-23.82°+\mathrm{p}.360°$$$$\theta =-11.91°+\mathrm{p}.180°\Rightarrow \mathrm{p}=1,2,3,...$$

Commented by tawa last updated on 31/Mar/17

$$\mathrm{God}\mathrm{bless}\mathrm{you}\mathrm{sir}.$$

Answered by ajfour last updated on 31/Mar/17

$$2\sin \theta \cos \theta +7(\cos {}^2\theta -\sin {}^2\theta )=6$$$$dividingby\cos {}^2\theta weget:$$$$2\tan \theta +7(1-\tan {}^2\theta )=6\sec {}^2\theta$$$$2\tan \theta +7-7\tan {}^2\theta =6+6\tan {}^2\theta$$$$13\tan {}^2\theta -2\tan \theta -1=0$$$$\tan \theta =\frac{2\pm \sqrt{4+52}}{26}$$$$\theta ={\tan }^{-1}\left(\frac{1\pm \sqrt{14}}{13}\right)+n\pi .$$

Commented by tawa last updated on 31/Mar/17

$$\mathrm{God}\mathrm{bless}\mathrm{you}\mathrm{sir}.$$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com