
Question and Answers Forum
Question Number 75435 by Crabby89p13 last updated on 11/Dec/19
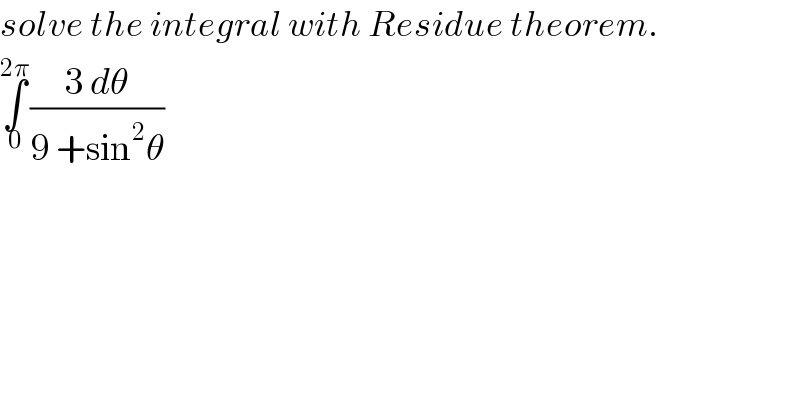
Commented by mathmax by abdo last updated on 11/Dec/19

Commented by Crabby89p13 last updated on 11/Dec/19

Commented by mathmax by abdo last updated on 11/Dec/19
Commented by Crabby89p13 last updated on 12/Dec/19

