
Question and Answers Forum
Question Number 174389 by Best1 last updated on 31/Jul/22
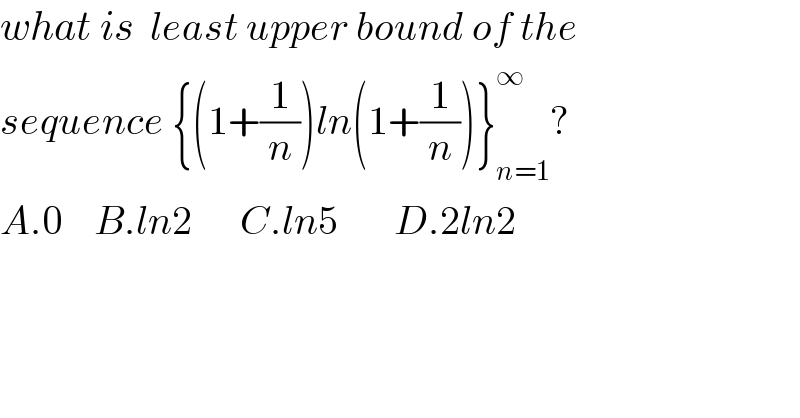
Answered by Gazella thomsonii last updated on 31/Jul/22
![Let′s think of a Sequence A_k Function as f(z) and Find Root f^((1)) (z)=0 for find inf [f(z),z_0 ] so , f(z)=(1+(1/z))ln(1+(1/z)) ((df(z))/dz)=−((1/z))^2 ln(1+(1/z))−((1+(1/z))/(1+(1/z)))∙((d )/dz)(1+(1/z))=0 ∴z_0 =−(e/(e−1)) inf[f(z),z=−(e/(e−1))]=lim_(x→z_0 ) f(z)=−(1/e) But, z∈Z^+ /{0} we don′t need inf[f(z)] when z is less than 0 inf[f(z),z=∞]=0 (becauselim_(z→∞) f(z)=0) sup is not Exist. (because lim_(z→0) f(z)=∞, f^((1)) (a)<0 ,a∈Z^+ /{0})](Q174392.png)
Commented by Best1 last updated on 31/Jul/22
| ||
Question and Answers Forum | ||
Question Number 174389 by Best1 last updated on 31/Jul/22 | ||
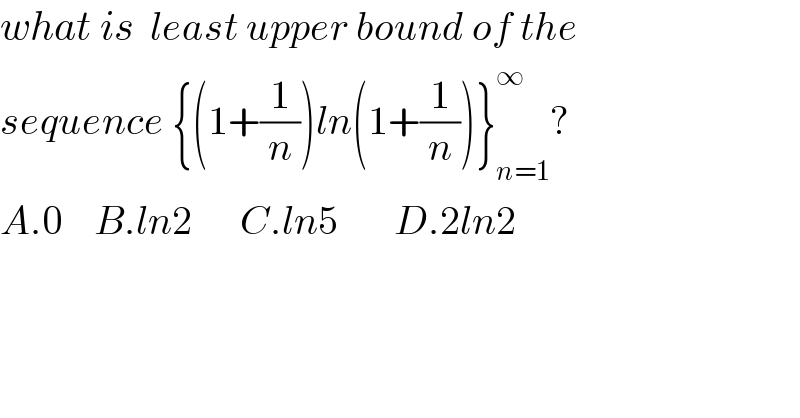 | ||
Answered by Gazella thomsonii last updated on 31/Jul/22 | ||
![Let′s think of a Sequence A_k Function as f(z) and Find Root f^((1)) (z)=0 for find inf [f(z),z_0 ] so , f(z)=(1+(1/z))ln(1+(1/z)) ((df(z))/dz)=−((1/z))^2 ln(1+(1/z))−((1+(1/z))/(1+(1/z)))∙((d )/dz)(1+(1/z))=0 ∴z_0 =−(e/(e−1)) inf[f(z),z=−(e/(e−1))]=lim_(x→z_0 ) f(z)=−(1/e) But, z∈Z^+ /{0} we don′t need inf[f(z)] when z is less than 0 inf[f(z),z=∞]=0 (becauselim_(z→∞) f(z)=0) sup is not Exist. (because lim_(z→0) f(z)=∞, f^((1)) (a)<0 ,a∈Z^+ /{0})](Q174392.png) | ||
| ||
Commented by Best1 last updated on 31/Jul/22 | ||
 | ||